Embarking on a journey through Section 1.3 using midpoint and distance formulas answer key, we unveil the intricate tapestry of geometric calculations, providing a comprehensive guide to unlocking the mysteries of line segments and points in space.
Delving into the depths of this mathematical realm, we will explore the fundamental concepts of midpoint and distance formulas, empowering you with the tools to navigate the complexities of geometry with precision and confidence.
1. Midpoint Formula
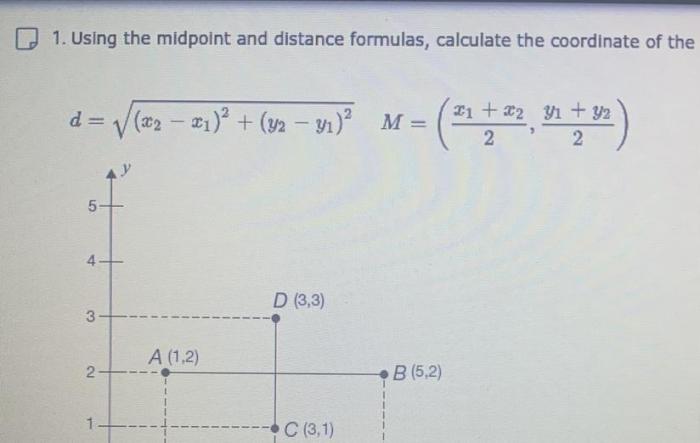
The midpoint formula calculates the midpoint of a line segment given the coordinates of its endpoints. It is defined as the average of the x-coordinates and the average of the y-coordinates of the endpoints.
Mathematically, if (x1, y1) and (x2, y2) are the coordinates of the endpoints, then the midpoint (x, y) is given by:
(x, y) = ((x1 + x2) / 2, (y1 + y2) / 2)
Examples, Section 1.3 using midpoint and distance formulas answer key
- Find the midpoint of the line segment with endpoints (2, 5) and (8, 11).
- ((2 + 8) / 2, (5 + 11) / 2) = (5, 8)
- Therefore, the midpoint is (5, 8).
Key Questions Answered: Section 1.3 Using Midpoint And Distance Formulas Answer Key
What is the midpoint formula?
The midpoint formula calculates the midpoint of a line segment given the coordinates of its endpoints. It is expressed as: Midpoint = ((x1 + x2) / 2, (y1 + y2) / 2), where (x1, y1) and (x2, y2) represent the coordinates of the endpoints.
How do I use the distance formula?
The distance formula determines the distance between two points in a plane. It is given by: Distance = sqrt((x2 – x1)^2 + (y2 – y1)^2), where (x1, y1) and (x2, y2) represent the coordinates of the two points.