Unit 5 test study guide systems of equations & inequalities – Introducing the Unit 5 Test Study Guide for Systems of Equations and Inequalities, a comprehensive guide designed to empower students with the knowledge and strategies to conquer this crucial topic. Delving into the intricacies of solving systems, graphing inequalities, and real-world applications, this guide will equip learners with the confidence to excel in their upcoming assessment.
Throughout this guide, we will explore the fundamental concepts of systems of equations, including substitution, elimination, and matrices. We will delve into the techniques for solving systems of inequalities, encompassing graphing, substitution, and elimination methods. Furthermore, we will uncover the practical applications of systems of equations and inequalities in various fields, demonstrating their relevance in science, engineering, and economics.
Systems of Equations
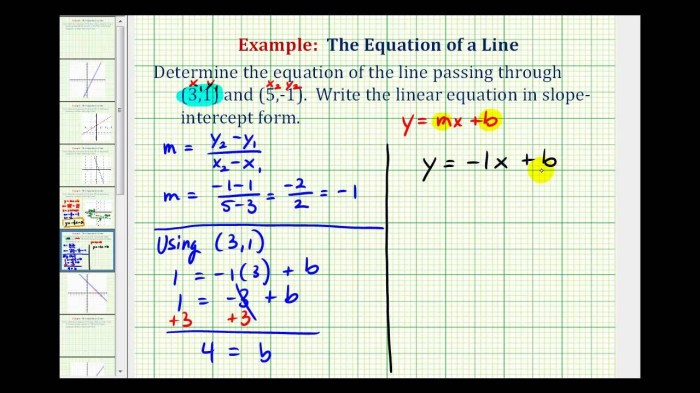
A system of equations is a set of two or more equations that have the same variables. Solving a system of equations means finding the values of the variables that satisfy all the equations simultaneously.
There are three main methods for solving systems of equations: substitution, elimination, and matrices. Substitution involves substituting the value of one variable from one equation into another equation. Elimination involves adding or subtracting the equations to eliminate one of the variables.
Matrices involve using matrix operations to solve the system of equations.
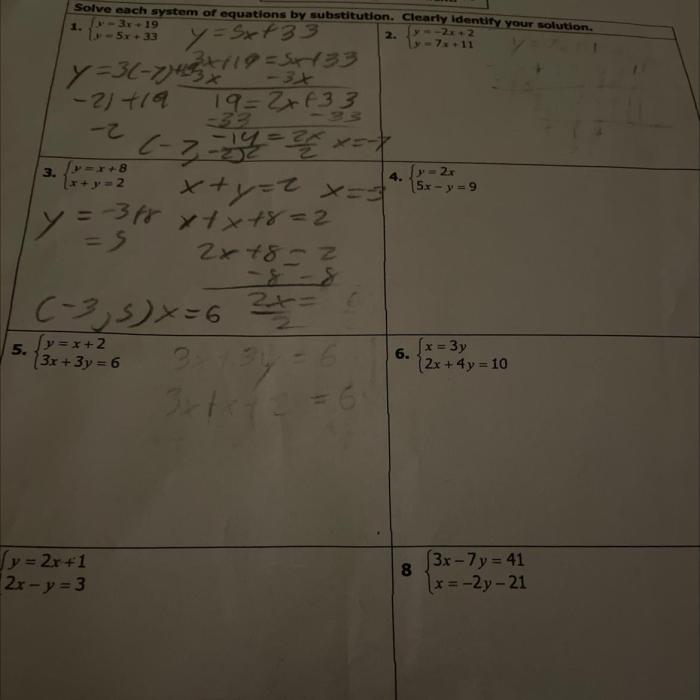
Solving Systems of Equations Using Substitution
- Solve one of the equations for one of the variables.
- Substitute the expression for that variable into the other equation.
- Solve the resulting equation for the other variable.
- Substitute the values of the variables back into the original equations to check your solution.
Solving Systems of Equations Using Elimination
- Add or subtract the equations to eliminate one of the variables.
- Solve the resulting equation for the remaining variable.
- Substitute the value of the variable back into one of the original equations to solve for the other variable.
- Check your solution by substituting the values of the variables back into the original equations.
Solving Systems of Equations Using Matrices
- Write the system of equations in matrix form.
- Use row operations to transform the matrix into an upper triangular matrix.
- Solve the upper triangular matrix for the variables.
- Check your solution by substituting the values of the variables back into the original equations.
Systems of Inequalities
A system of inequalities is a set of two or more inequalities that have the same variables. Solving a system of inequalities means finding the values of the variables that satisfy all the inequalities simultaneously.
There are two main methods for solving systems of inequalities: graphing and substitution. Graphing involves graphing each inequality and finding the region that satisfies all the inequalities. Substitution involves substituting the value of one variable from one inequality into another inequality.
Graphing Systems of Inequalities
- Graph each inequality on the same coordinate plane.
- Shade the region that satisfies all the inequalities.
- The solution to the system of inequalities is the region that is shaded by all the inequalities.
Solving Systems of Inequalities Using Substitution
- Solve one of the inequalities for one of the variables.
- Substitute the expression for that variable into the other inequality.
- Solve the resulting inequality for the other variable.
- Substitute the values of the variables back into the original inequalities to check your solution.
Applications of Systems of Equations and Inequalities: Unit 5 Test Study Guide Systems Of Equations & Inequalities
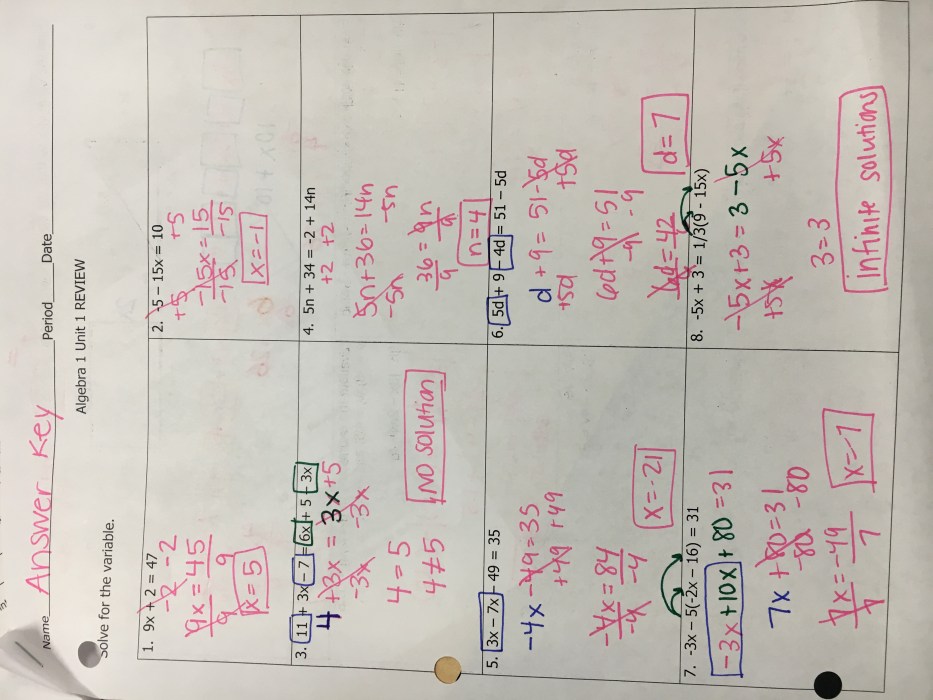
Systems of equations and inequalities have many applications in real-world problems. For example, they can be used to:
- Model and solve problems in science, engineering, and economics.
- Find the intersection or union of two or more sets of data.
- Determine the feasibility of a given solution.
- Make predictions and forecasts.
Example Applications, Unit 5 test study guide systems of equations & inequalities
- In science, systems of equations can be used to model the motion of objects, the flow of fluids, and the chemical reactions.
- In engineering, systems of equations can be used to design structures, bridges, and machines.
- In economics, systems of equations can be used to model the supply and demand of goods and services.
- Read the instructions carefully and make sure you understand what is being asked.
- Start by solving the easiest problem first.
- Show all your work, even if you make a mistake.
- Check your answers before you submit your test.
- Don’t spend too much time on any one problem.
- If you get stuck, move on to the next problem and come back to it later.
- Use your time wisely and don’t waste time on problems that you don’t know how to solve.
- Read the problem carefully and make sure you understand what is being asked.
- Write down the given information and any formulas that you need to use.
- Show all your work, even if you make a mistake.
- Check your answers before you submit your test.
Test-Taking Strategies
Here are some effective test-taking strategies for systems of equations and inequalities:
Tips for Managing Time
Tips for Minimizing Errors
FAQ Summary
What are the different methods for solving systems of equations?
Substitution, elimination, and matrices
How do you graph a system of inequalities?
Plot the boundary lines of each inequality and shade the region that satisfies all inequalities
What are some real-world applications of systems of equations and inequalities?
Mixing chemicals, designing circuits, and optimizing profits